Gas stoichiometry: Difference between revisions
imported>Milton Beychok m (Creating anew article in progress) |
imported>Milton Beychok m (→Example calculations: Wiki link) |
||
| Line 17: | Line 17: | ||
==Example calculations== | ==Example calculations== | ||
Gas stoichiometry calculations solve for the unknown [[volume]] or [[mass]] of gaseous products and/or reactants involved in chemical reactions by using, for the most part, the [[ideal gas law]].<ref>{{cite book|author=John Olmstead III and Gregory M. Williams|title=Chemistry: The Molecular Science|edition=2nd Edition|publisher=Wm. V. Brown|year=1997|pages=p. 229|id=ISBN 0-8151-8450-6}}</ref><ref>{{cite book|author=Steven S. Zumdahl|title=Chemical Principles|edition=6th Edition|publisher=Houghton Mifflin|year=2007|pages=p.150|id=ISBN 0-547-0048-7}}</ref> | Gas stoichiometry calculations solve for the unknown [[Volume (science)|volume]] or [[mass]] of gaseous products and/or reactants involved in chemical reactions by using, for the most part, the [[ideal gas law]].<ref>{{cite book|author=John Olmstead III and Gregory M. Williams|title=Chemistry: The Molecular Science|edition=2nd Edition|publisher=Wm. V. Brown|year=1997|pages=p. 229|id=ISBN 0-8151-8450-6}}</ref><ref>{{cite book|author=Steven S. Zumdahl|title=Chemical Principles|edition=6th Edition|publisher=Houghton Mifflin|year=2007|pages=p.150|id=ISBN 0-547-0048-7}}</ref> | ||
===Acetylene and hydrogen produced by cracking methane=== | ===Acetylene and hydrogen produced by cracking methane=== | ||
Revision as of 22:35, 20 June 2010
Gas stoichiometry is the quantitative relationship between the reactants and products in chemical reactions that produce gases. Gas stoichiometry applies when all of the gases involved in a chemical reaction (either reactants or products) may be assumed to be ideal, and the temperature and pressure of the gases are all known. Often, but not always, the reference conditions used for gas stoichiometric calculations are taken to be a temperature of 0 °C and an absolute pressure of 1 atm.
History
In 1792, the German chemist Jeremias B. Richter first proposed the concept of the quantitative mass relationships that exist between the chemicals involved in chemical reactions:[1]
In German: Die stöchyometrie ist die Wissenschaft die quantitativen oder Massenverhältnisse zu messen, in welchen die chymischen Elemente gegen einander stehen." (In English: Stoichiometry is the science of measuring the quantitative proportions or mass ratios in which chemical elements stand to one another.)[2]
He named this quantitative study of chemistry to be "stoichiometry", from two Greek words meaning to measure the magnitude of something that cannot be divided.[3]
Richter's introduction of the term "stoichiometry" substantially predated the introduction of the atomic hypothesis in 1803 by the English eminent scientist John Dalton.
Example calculations
Gas stoichiometry calculations solve for the unknown volume or mass of gaseous products and/or reactants involved in chemical reactions by using, for the most part, the ideal gas law.[4][5]
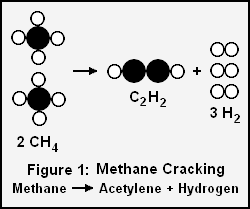
Acetylene and hydrogen produced by cracking methane
For example, if we want to determine the volume of acetylene and hydrogen gases produced by cracking 100 grams of methane gas as per this chemical reaction:
- 2 CH4 → C2H2 + 3H2
- 2 moles of methane gas → 1 mole of acetylene gas + 3 moles of hydrogen gas
Determining the moles of methane (molar mass = 16.04 g/mol) equivalent to 100 grams :
There is a 2 to 1 molar ratio of methane (CH4) to product acetylene (C2H2) in the above cracking reaction, so 3.117 moles of acetylene will be formed.
The ideal gas law and the universal molar gas constant of R = 8.205745 × 10 −5 m3 atm K −1 mol −1 can now be used to solve for the volume of acetylene at 0 °C (273.15 K) and 1 atmosphere:
The molar ratio of methane to product hydrogen (H2) in the above cracking reaction is 1 to 1.5, so 9.351 moles of hydrogen will be formed. Again using the ideal gas law, the volume of hydrogen formed at 0 °C (273.15 K) and 1 atmosphere will be:
Since the molar mass of acetylene and of hydrogen are 26.036 g/mol and 2.0158 g/mol respectively, the mass of acetylene formed is 26.036 × 3.117 = 81.15 g and the mass of hydrogen formed is 2.0158 × 9.351 = 18.85 g. The total mass of products is then 81.15 + 18.85 = 100.00 g which is equal to the mass of the reactant methane, and that satisfies the law of conservation of mass.
The parameters in the above equations are:
| = absolute gas pressure, in atm | |
| = gas volume, in m3 | |
| = number of moles, in mol | |
| = absolute gas temperature, in K | |
| = molar mass of gas, in g/mol | |
| = 8.205745 × 10 −5 = universal molar gas constant, in m3 atm K −1 mol −1 |
Nitrogen and hydrogen required for ammonia synthesis
As another example, if we want to determine the amounts of nitrogen and hydrogen gases required to produce 5 kg of ammonia gas per this chemical reaction for the synthesis of ammonia:
- N2 + 3 H2 → 2 NH3
- 1 mole of nitrogen gas + 3 moles of hydrogen gas → 2 moles of ammonia gas
Determining the moles of ammonia (molar mass = 17.03 g/mol) equivalent to 5 kilograms:
There is a 2 to 1 molar ratio of product ammonia (NH3) to reactant nitrogen (N2) in the above synthesis reaction, so 146.80 moles of reactant nitrogen will be required.
The ideal gas law and the universal molar gas constant can again be used to solve for the volume of nitrogen at 0 °C (273.15 K) and 1 atmosphere:
There is a 3 to 1 molar ratio of reactant hydrogen (H 2) to reactant nitrogen in the above synthesis reaction, so 440.40 moles of reactant hydrogen will be required. Thus:
Note that in this example, as well as the previous example, that volume ratios are equal to molar ratios.
Since the molar mass of nitrogen and of hydrogen are 28.0134 g/mol and 2.0158 g/mol respectively, the mass of nitrogen formed is 28.0134 × 146.80 = 4,112 g and the mass of hydrogen formed is 2.0158 × 440.40 = 888 g. The total mass of products is then 4,112 + 888 = 5,000 g = 5 kg which is equal to the mass of the reactant methane, and that satisfies the law of conservation of mass.
References
- ↑ J. B. Richter (1792-1794). Anfangsgründe der Stöchyometrie oder Messkunst chymischer Elemente. Johann Friedrich Korn, Breslau and Hirschberg, Germany. Available online at Google Books
- ↑ Johann Bartholomäus Trommsdorff (1795). Journal der Pharmacie für Ärzte und Apotheker, Volume 2, Leizig, Germany, p. 269. Available online at Google Books
- ↑ Some Stoichiometric Musing From the website of the Illinois State University's chemistry department.
- ↑ John Olmstead III and Gregory M. Williams (1997). Chemistry: The Molecular Science, 2nd Edition. Wm. V. Brown, p. 229. ISBN 0-8151-8450-6.
- ↑ Steven S. Zumdahl (2007). Chemical Principles, 6th Edition. Houghton Mifflin, p.150. ISBN 0-547-0048-7.